TY - JOUR T1 - Convergence of an Embedded Exponential-Type Low-Regularity Integrators for the KdV Equation Without Loss of Regularity AU - Li , Yongsheng AU - Wu , Yifei AU - Yao , Fangyan JO - Annals of Applied Mathematics VL - 1 SP - 1 EP - 21 PY - 2021 DA - 2021/02 SN - 37 DO - http://doi.org/10.4208/aam.OA-2020-0001 UR - https://global-sci.org/intro/article_detail/aam/18628.html KW - The KdV equation, numerical solution, convergence analysis, error estimate, low regularity, fast Fourier transform. AB -

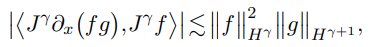
In this paper, we study the convergence rate of an Embedded exponential-type low-regularity integrator (ELRI) for  the  Korteweg-de Vries equation. We develop some new harmonic analysis techniques to handle the "stability" issue. In particular, we use a new stability estimate which allows us to avoid the use of the fractional Leibniz inequality,

image.png

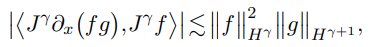
and replace it by suitable inequalities without loss of regularity. Based on these techniques, we prove that the ELRI scheme proposed in [41] provides $\frac12$-order convergence accuracy  in $H^\gamma$ for any initial data belonging to $H^\gamma$ with $\gamma >\frac32$, which  does not require any additional derivative assumptions.