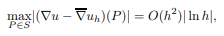
We study the gradient superconvergence of bilinear finite volume
element (FVE) solving the elliptic problems. First, a superclose
weak estimate is established for the bilinear form of the FVE
method. Then, we prove that the gradient approximation of the FVE
solution has the superconvergence property:
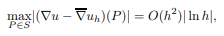
where 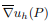 denotes the average gradient on elements
containing point $P$ and $S$ is the set of optimal stress points
composed of the mesh points, the midpoints of edges and the centers of elements.
denotes the average gradient on elements
containing point $P$ and $S$ is the set of optimal stress points
composed of the mesh points, the midpoints of edges and the centers of elements.